This data-file calculates the energy stored in rotating masses. This includes 50Wh – 100 kWh flywheels. In power grids, the angular momentum of large rotating generators is known as ‘inertia’ and can run to 30kWh to 30MWH per turbine. Inertia has historically been a crucial part of stabilizing grids. And the world’s “original” energy storage device. Hence how do the physics work? And how is the energy of a rotating object calculated?
Rotational energy: how do the mechanical physics work?
Linear motion. Newton’s Second Law, familiarly, tells us that F = ma, for linear acceleration a. And by extension, the kinetic energy of a moving object E = ½ m(V^2). Where E = energy (Joules), m = mass (kg) and v = velocity (meters/second).
The same laws can be adapted for rotational acceleration, stating that F = Iα. And by extension, the kinetic energy of a rotating object E = ½ I (ω^2) where ω is rotational velocity. And α is angular acceleration, the rotund cousin of linear acceleration, a. E = energy (Joules), I is the ‘moment of inertia’ around the axis of rotation (in kg-m2) and ω is rotational velocity (in radians per second).
These units are admittedly a bit weirder. 1 radian is 57.296°, so an angular velocity of 1 radian per second means a circular object turns by 57.296° around its axis each second. It does not matter how wide the diameter is. 1 radian per second means it will take 6.3 seconds to do a full turn.
Moment of Inertia is to rotating objects what mass is to objects that are moving in a straight line. Moment of Inertia can be captured via equations. For example: I = k M (R^2). Where I is moment of Inertia (kg-m2), M is the mass of the object (kg), R is the radius (m). Finally k is a “shape-based scalar”, which integrates the way in which the mass is distributed across different distances from the center of rotation. k depends on shape. But generally, k=0.5 for a disc or horizontal rod. k=1 for a wheel that is loaded at the rim. k = 2/5 for a solid sphere, 2/3 for a spherical shell.
All of this is calculated for you in the data-file. And for more details on converting one energy unit into another, please see our overview of energy units.
Rotational energy: the world’s original battery?
The ability of rotating objects to store and smooth energy flows has been known for around 8,000 years. Flywheels were used in the manufacture of pottery in China and Mesopotamia since 6 000 BCE.
In medieval times, the spinning wheel started displacing hand spindles from around 1,200 AD, unlocking an order of magnitude improvement in the rate of spinning wool into yarns and threads. Closer to home, the sewing machine below is in my in-laws’ house, and I have done my best not to break it…
While these small energy storage devices are useful in smoothing out the jerky motion of human arms and legs, they do not store very much energy, maybe around 0.01 to 0.1 Wh. This is due to their small size (<30cm radiuses) and slow rotation speeds (<250 rpm).
Industrial Revolution: Next Came the Steam Era !
There is nothing fundamentally different between the sewing machine shown above and the large steam turbines that defined the nineteenth century. This might sound surprising, but bear with us…
The sewing machine operator must use their internal sense of rhythm (mine clearly being very poor, from the video above!), to press down with their leg, at the exact right time to add energy into the flywheel. Cleverly, in the steam engine’s case, the timing is controlled mechanically by a series of valves that are connected to the flywheel. Steam is introduced into a chamber at one part of the cycle, its pressure builds up, then the pressure is released into a piston, to add energy into the flywheel at the exact right moment.
What clearly did change with the advent of steam engines is that a lot more energy could be stored in these giant flywheels, harvesting the thermal energy of coal, rather than the limited energy of muscles.
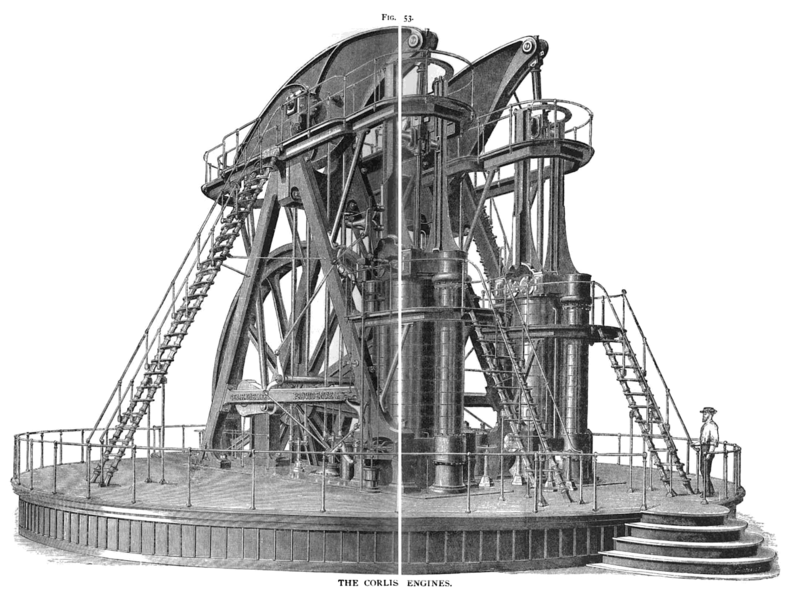
Possibly the largest steam engine ever built was the Corliss Centennial Engine, constructed for the Centennial Exposition, in Philadelphia, in 1876 and pictured below. It was quite possibly the first ever MW-scale combustion turbine, at 1,400 hp (1,045 kW). With some rough maths, we think the flywheel stored around 2-3kWh of energy, as a 9m wide iron wheel, weighing 100 tons rotated at 36 rpm.
Fast-forward: the largest rotating generators in the world?
The average nuclear plant in the grid today has 1.1 GW of capacity, the average coal plant is 400MW and the average natural gas plant is 100 MW. These are enormous numbers. And they impart an enormous amount of ‘inertia’ to grids.
The concept of inertia is all explained in our note below, which covers power generation from first principles. And if that isn’t nerdy enough, there is a whole follow-up note on the related and equally important topic of reactive power compensation.
Back to rotational energy, consider that one of the largest rotating turbines in the world is HPC’s 1,770MW Arabelle turbine. This is 7m in diameter, 17m long, weighs 4000 tons and spins at 1500 rpm. It could conceivably store almost 30 MWH of energy simply via the angular momentum of its massive rotor. This is huge for power quality and power grids.
Flywheels as a modern source of energy storage?
Flywheels can be used as an energy storage device, directly in mechanical contexts (echoing the sewing machines and spinning wheels of the past), to add inertia into power grids, or to recuperate energy that would otherwise be lost. Some interesting examples are below.
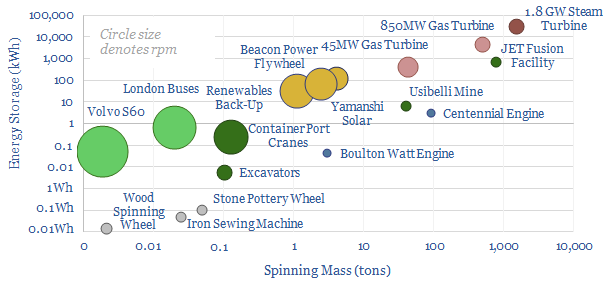
Yamanshi Solar. In 2015, Japan built the world’s largest super-conducting flywheel, to back up a 1MW solar installation. We think the flywheel has about 110kWh of rotational energy storage.
Likewise, in the US, Beacon Power has pioneered the use of flywheels for frequency regulation, with 20 MW plants located in Stephentown, New York and Hazel Township, Pennsylvania. The company is paid by the grid for providing frequency regulation as a service.
JET is the Joint European Torus Research Facility in Oxford, requiring rapid bursts of energy to test nuclear fusion. It came up in our research note in the frontiers of nuclear fusion. In order to provide the enormous bursts of energy to instigate nuclear fusion reactions, it has 2 giant flywheels. Each one is ramped up over 9-minutes using 8.8MW motors, then the system discharges 700 kWh of energy at a peak power of 500 MW.
The data-file also includes mining assets and London buses, which can recuperate up to 70% of the energy from braking or lowering a dragline under gravity, by shunting the energy into speeding up a flywheel. This might save 20-30% of the energy needed to re-accelerate the bus or raise the dragline again.
There is more on flywheels, supercapacitors, batteries and smoothing out renewables volatility via the links in this sentence.
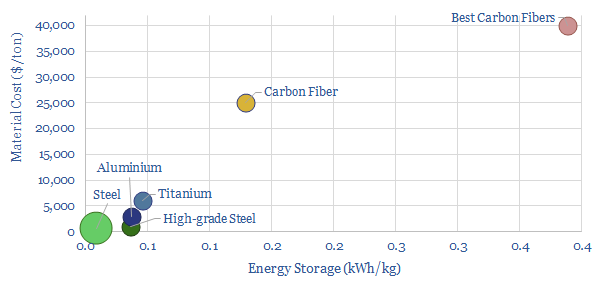
Materials matter: high-strength low-weight maximizes energy storage?
Our equations above showed that more energy is stored by a rotating object, when it rotates faster (square function), when it is heavier (linear), and when the weight is distributed further from the center of rotation (>square function).
However, there is a limit. The stress exerted on a material also rises as a square function of its rotational speed and a square function of its radius. So for example a steel disc of 1m diameter and 420 MPa of yield strength cannot be spun faster than 7,000 rpm before it is at risk of breaking.
As a result, there is also a growing movement to use high-performance materials in flywheels. Ultimately, a material such as carbon fiber can likely be spun 2-4x faster than steel wheels. While the disc cost rises linearly, the total system cost is deflated. This adds to the use of vacuum chambers and magnetically levitating bearings. The physics of flywheels are interesting. Please download the data-file for more.