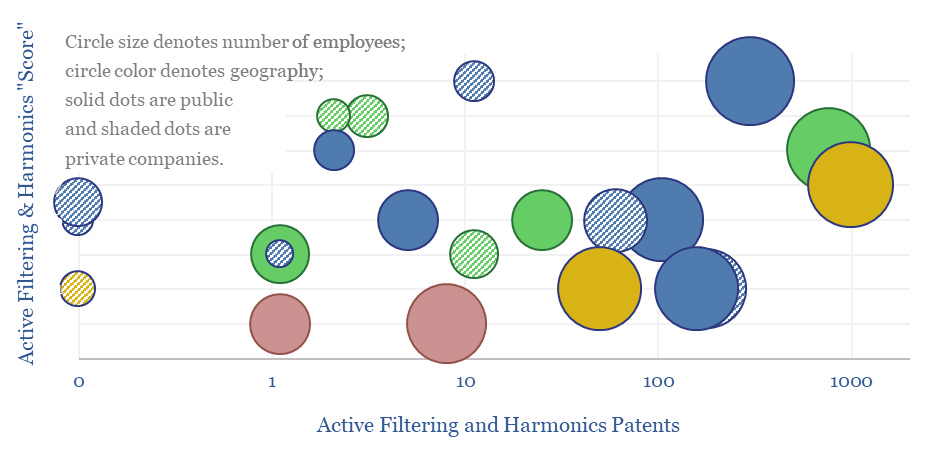
This data-file screens 20 leading companies in harmonic filters, tabulating their size, geography, ownership, patent filings and a description of their offering. Active harmonic filters reduce total harmonic distortion below 5%, with 97% efficiency, within 5 ms. Half a dozen companies stood out in our screen, including one large, listed Western capital goods company.
Power quality is increasingly important, as electricity demand grows, placing greater reliance on the power grids, which also increasingly feature non-linear loads such as variable frequeny drives, LEDs, EV chargers, data-centers, heat pumps, and inverter-based generation sources such as solar and wind. An introduction to power quality issues is presented in our overview of electricity.
Active harmonic filters are the most comprehensive method for avoiding power quality issues associated with harmonics, detecting harmonics in real time, then injecting opposing harmonics, in order to achieve a sinusoidal waveform, typically reducing total harmonic distortion below 5%, with 97% efficiency, within around 5 ms.
Hence this data-file screens 20 leading companies in harmonic filters, tabulating their size, geography, ownership details, patent filings and a description of their offering. The screen includes companies listed in the US, Europe, Japan and private companies. The landscape also includes a large degree of consolidation recently.
The costs of active harmonic filters are also tabulated in a backup tab, and can range from $100/kVA to $1,600/kVA, depending on the degree of harmonic filtering, responsiveness and efficiency.
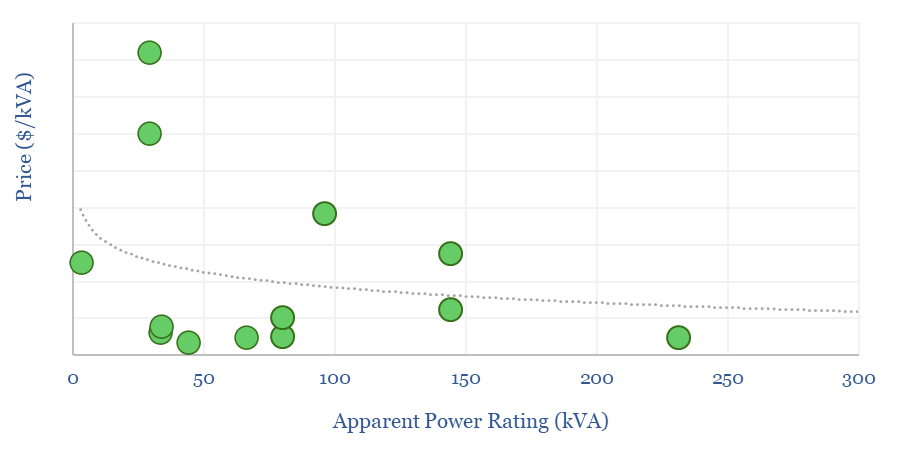
We found that one large, listed Western capital goods company seems to have a leading offering in active harmonic filters, which is also reflected in premium pricing for its active filters in the chart above.
Another backup tab summarizes the details of some excellent case studies from Comsys, which is one of the leading companies in harmonic filters, screened in the data-file.
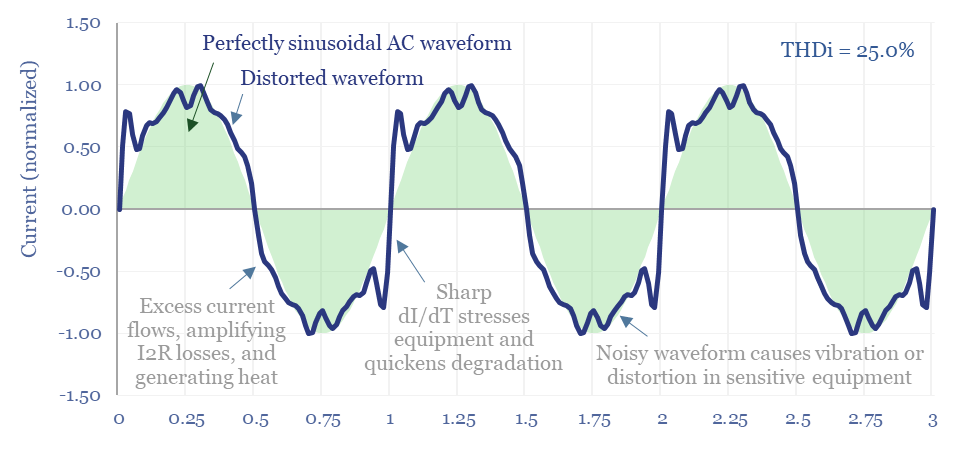
Another backup tab in the data-file computes total harmonic distortion based on a Fourier decomposition of AC waveforms, in order to chart the resultant waveform, after adding harmonics up to the 20th order.
Finally, another backup tab features our notes from technical papers and case studies into avoiding harmonics.